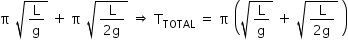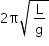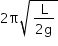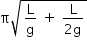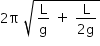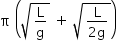Um pêndulo simples, constituído por um fio de comprimento L e uma pequena esfera, é colocado em oscilação. Uma haste horizontal rígida é inserida perpendicularmente ao plano de oscilação desse pêndulo, interceptando o movimento do fio na metade do seu comprimento, quando ele está na direção vertical. A partir desse momento, o período do movimento da esfera é dado por
Note e adote:
A aceleração da gravidade é g.
Ignore a massa do fio.
O movimento oscilatório ocorre com ângulos pequenos.
O fio não adere à haste horizontal.
A situação descrita no enunciado pode ser representada pelo esquema a seguir:
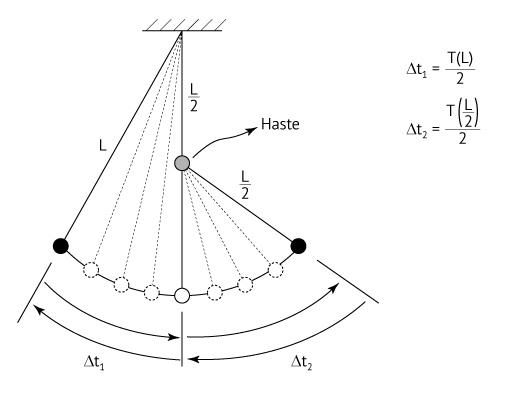
- Período do pêndulo (TTOTAL) descrito no enunciado:
TTOTAL = Δt1 + Δt2
- Cálculo de Δt1 =
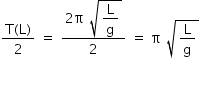
- Cálculo de Δt2 =
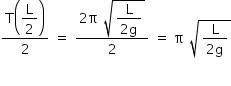
TTOTAL =