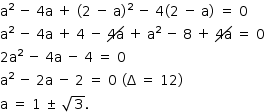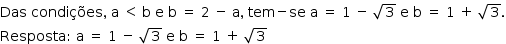Sejam c um número real e f(x) = x 2 - 4x + c uma função quadrática definida para todo número real x. No plano cartesiano, considere a parábola dada pelo gráfico de y = f(x).
a) Determine c no caso em que a abscissa e a ordenada do vértice da parábola têm soma nula e esboce o respectivo gráfico para 0 ≤ x ≤ 4.
b) Considere os pontos de coordenadas A = (a, f(a)) e B = (b,f (b)), onde a e b são números reais com a < b. Sabendo que o ponto médio do segmento ̅ , determine a e b.
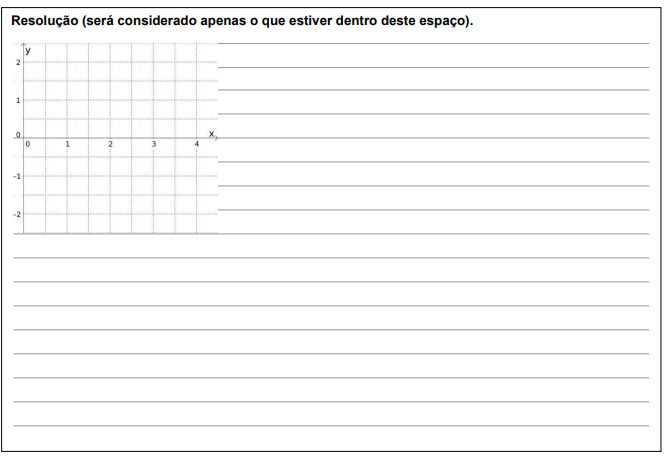
a) A abscissa do vértice da parábola da equação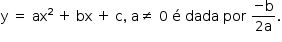 
Com y = x2 – 4x + c, essa abscissa é 2 (=xv). A ordenada do vértice da parábola y = x2 – 4x + c é dada por 22 – 4 · 2 + c, ou seja, c – 4 (=yv). De xv + yv = 0, tem-se 2 + c – 4 = 0, ou seja, c = 2.
Resposta:
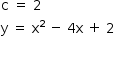
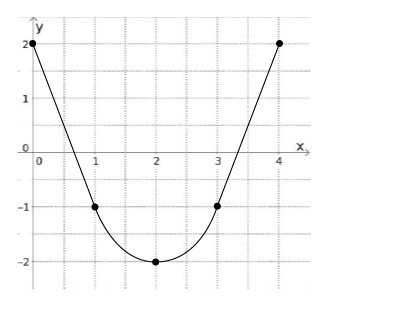
| x | y |
|---|---|
| 0 | 2 |
| 1 | –1 |
| 2 | –2 |
| 3 | –1 |
| 4 | 2 |
b) M(1, c) é o ponto médio de A(a, f(a)) e B(b, f(b))
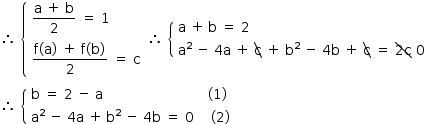
De (1) e (2), tem-se:
