A - Represente graficamente no plano cartesiano a função: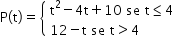 
Se a função P(t ) , em centenas de reais, expressa o preço de um produto depois de estar t anos no mercado (0 ≤ t ≤ 8), qual foi o preço máximo alcançado pelo produto?
B - Qual foi o menor preço alcançado pelo produto nesse período de 8 anos?
A)
Para construir o gráfico de uma função definida por mais de uma sentença, analisa-se cada sentença separadamente.
I) O gráfico da função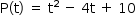 
, para 
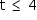, é o arco de parábola representado a seguir:
II) O gráfico da função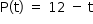 
, para 
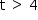, é a semirreta representada a seguir:
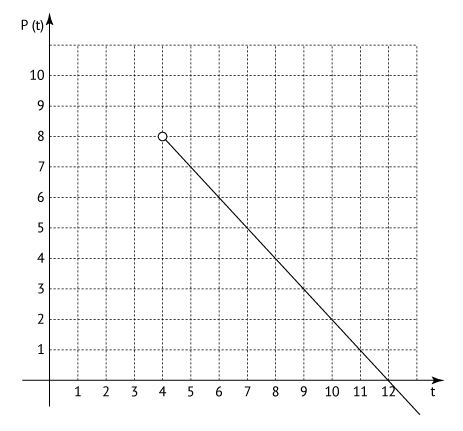
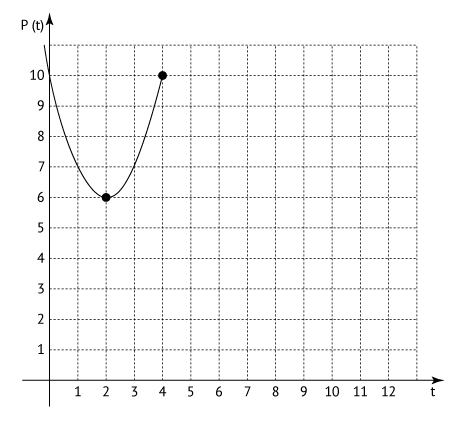
A reunião dos gráficos obtidos em (I) e (II) é o gráfico da função P(t):
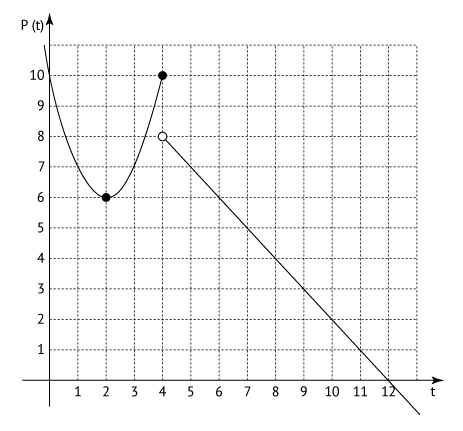
Restringindo-se o gráfico ao intervalo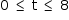 
, tem-se:
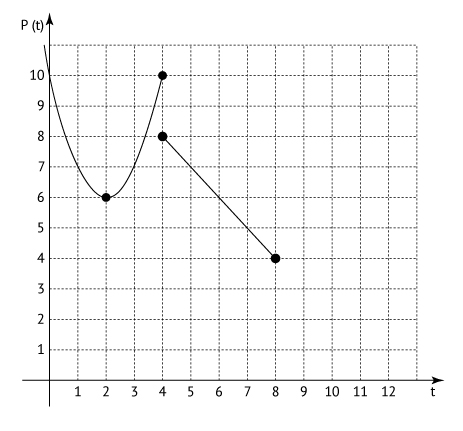
Dessa representação gráfica, pode-se observar que o preço máximo alcançado pelo produto no intervalo 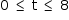é de 10 centenas de reais, ou seja, R$ 1000,00.
Resposta:
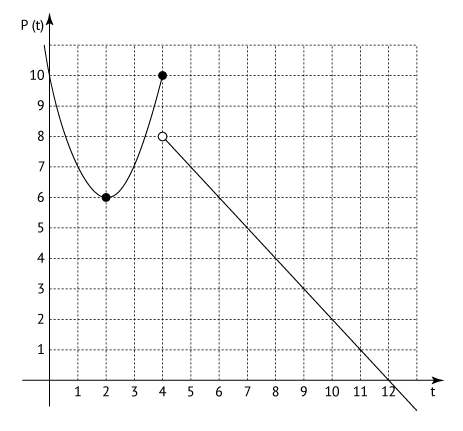
e R$ 1000,00.
B)
Do item anterior, pela representação gráfica tem-se que o menor preço alcançado pelo produto no intervalo 0 ≤ t ≤ 8 é de 4 centenas de reais, ou seja, R$ 400,00.
Resposta: R$ 400,00.